

I see that Abhishek009 gave you a very thoughtful response.
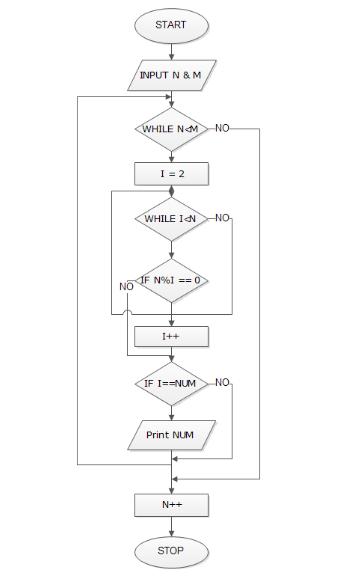
If-n-is-a-positive-integer-which-of-the-following-must-be-true-217202.html I added the same question from E-gmat forum too. I believe every prime number that exists on the planet can be written either as 4N+1 or as 4N-1 I however disagree with the statement regarding 4N+1 and 4N-1 There's no zero option in the question, so this must be a prime number.Įxcellent Solution and i will be using this in the future questions We can eliminate this from the list also. We know that 22 is a multiple of 11, so 220 is also a multiple of 11. We've already checked all the prime numbers less than 10, so we know that neither of these numbers is divisible by anything less than 10. Those two numbers are also multiples of 7, so eliminate them from the list. Now, notice that a cool thing about this range is that 210 is also a multiple 7 (again, because 21 is a multiple of 7). When we eliminate those, we are left with. If we add or subtract 3 or 9, we get more multiples of three. Notice that 21 is a multiple of 3, so 210 is also a multiple of 3. That leaves us with:Įliminate the four multiples of 3. First, eliminate all the even numbers and the odd multiples of 5 in that range. The 6N+1 or 6N-1 rule is basically every odd number that is not divisible by three, so it narrows the search a little. That's not a rule unique to prime numbers at all. If you divide any odd number by 4, you will get a remainder of either 1 or 3. My friend, every single odd number greater than can be written either as 4N+1 or as 4N+3. Looking forward to your solution too mikemcgarry To find if a number is prime or not we need to check the divisibility with prime numbers less than the square root of that number. I checked every value for divisibility with prime numbers less than 15 (given that 15^2=225 and all values are less than 225 and above 200 Here the rule i used is well I didnt use any rule. Fun fact => every integer (>5) which is prime can also be written as either 4N+1 or 4N+3


 0 kommentar(er)
0 kommentar(er)
